JEE 2016 :: Advanced 2016 :: Physics 2016

• Advanced 2016 - Physics 2016
• Advanced 2016 - Chemistry 2016
• Advanced 2016 - Mathematics 2016

The ends Q and R of two thin wires, PQ and RS, are soldered (joined) together. Initially, each of the wire has a length of 1m 10⁰ C. Now, the end P is maintained at 10° C, While the end S is heated and maintained at 400° C. The system is thermally insulated from its surroundings. If the thermal conductivity of wire PQ is twice that of the wire RS and the coefficient of linear thermal expansion of PQ is  $1.2 \times 10^{-5}K^{-1}$, the change in length of the wire PQ is

Answer:

Explanation:

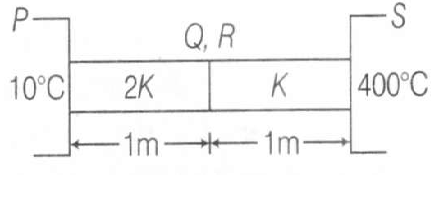
Rate of heat flow from P to Q,

$\frac{\text{d}Q}{\text{d}t}= \frac{2KA(T-10)}{1}$

 Rate of heat flow from Q to S

$\frac{\text{d}Q}{\text{d}t}= \frac{KA(4000-T)}{1}$

At steady state, state rate of heat flow is same

$\therefore \frac{2KA(T-10)}{1}= KA(400-T)$

or  2T-20=400-T  or 3T=420

    T= 140°

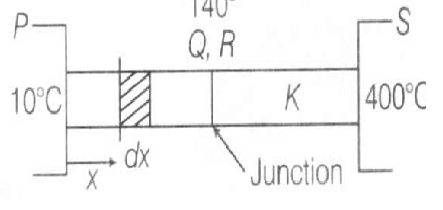
The temperature of the junction is 140° C.

Temperature at  a distance x from end P is T_x = (130 x+10°)

 Change in lenght dx is suppose dy

Then,  $dy=\propto dx(T_{x}-10)$

$\int_{0}^{\triangle y} dy= \int_{0}^{1} \propto dx(130x+10-10)$

$\triangle y=[\frac{\alpha x^{2}}{2}\times130]_0^1$

$\triangle y=1.2\times 10^{-5}\times 65$

$\triangle y= 78 \times 10^{-5}m=0.78 m$

A gas is enclosed in a cylinder with a movable frictionless piston. Its initial thermodynamic state at pressure $P_{i}=10^{5}$ P_a  and volume $V_{i}=10^{-3}m^{3}$ changes to final state at  $P_{f}=(\frac{1}{32})\times 10^{5} P_{a}$  and  $V_{f}=8 \times 10^{-3} m^{3}$ in an adiabatic  quasi-static  process, such  that $ P^{3} V^{5}$ =  constant. Consider another thermodynamic process that brings the system from the same initial state to the same final state in two steps : an isobaric expansion at $P_{i}$  followed by an isochoric(isovolumetric) process at  volume $V_{f}$ . The amount of heat supplied to the system  in the two step process is approximately

Answer:

Explanation:

In the first process $p_{i}V_{i}^{\gamma}=p_{f}V_{f}^{\gamma}$

$\Rightarrow \frac{p_{i}}{p_{f}}=(\frac{V_{f}}{V_{i}})^{\gamma}\Rightarrow32=8^{\gamma}$

$\gamma =\frac{5}{3}$ ......(i)

 For the two step process

$W= P_{i}(V_{f}-V_{i})=10^{5}(7\times 10^{-3})$

$W= 7\times 10^{2}$ J

$\triangle U= \frac{f}{2}(p_{f}V_{f}-p_{i}V_{i})$

$=\frac{I}{\gamma-1}(\frac{1}{4}\times 10^{2}-10^{2})$

$\triangle U= -\frac{3}{2}.\frac{3}{4}\times 10^{2}=-\frac{9}{8}\times 10^{2}J$

$Q-W=\triangle U$

$\Rightarrow 7\times 10^{2}-\frac{9}{8}\times 10^{2}$

$=\frac{47}{8}\times 10^{2}J =588J$

The electrostatic energy of Zprotons uniformly distributed throughout a spherical nucleus of radius R is given by $E=\frac{3}{5}\frac{Z(Z-1)e^{2}}{4\pi\epsilon_{0}R}$.The measured masses of the neutron, $_{1}^{1}H,_{7}^{15}N $and $ _{8}^{15}O $are  1.008665u. 1.007825u, 15.000109u and 15.003065 u. respectively. Given that the radii of both the $_{7}^{15}N $ and$ _{8}^{15} O $ nuclei are same,1 u =931.5MeV/c² ,c is the speed of light and $e^{2}/(4\pi \epsilon_{0})=1.44$ meV fm.Assuming that the difference between the binding energies of $_{7}^{15}N $ and $_{8}^{15}O$  is purely due to the electrostatic energy, the radius of either of the nuclei is (1 fm = 10^-15m)

Answer:

Explanation:

Electrostatic energy = Binding energy of N - Binding energy of O

=[(7M_H+8M_n-M_N)]-[(8M_H+7M_n-M_O)]xc²

=[-M_H+M_n+M_o-M_N]c²

=[- 1.007825 + 1.008665 + 15.003065- 15.000109]x 931.5 = + 3.5359 MeV

$  \triangle E=\frac{3}{5}\times \frac{1.44\times 8\times7}{R}-\frac{3}{5}\times \frac{1.44\times 7\times 6}{R}=3.5359 MeV$

$R=\frac{3\times 1.44\times 14}{5\times 3.5359}=3.42 fm$

An accident in a nuclear laboratory resulted in deposition of a certain amourt of radioactive material of half-life 18 days inside the laboratory. Tests revealed that the radiation was 64 times more than the permissible level required for safe operation of the laboratory. What is the minimum number of days after which the laboratory can be considered safe for use?

Answer:

Explanation:

Using the relation

$R=R_{0}\left(\frac{1}{2}\right)^{n}$

Here. R is activity of radioactive substance, R₀  initial activity and n is number of half-lives.

$1=64\left(\frac{1}{2}^{}\right)^{n}$

Solving  we get, n=6

Now ,t =n(t_1\2)=6(18 days)=108 days

A metal is heated in a furnace where a sensor is kept above the metal surface to read the power radiated (P) by the metal. The sensor has a scale that displays \log(P/P_{0}) where P₀ is a constant. When the metal surface is at a temperature of 487⁰C, the sensor shows a value 1. Assume that the emissivity of the metallic surface remains constant. What is the value displayed by the sensor when the temperature of the metal surface is raised to 2767⁰C?

Answer:

Explanation:

  $\log_{2}\frac{P_{1}}{P_{0}}=1$

Therefore $\frac{P_{1}}{P_{2}}=2$

According Stefan's law $p\proptoT$

  $\frac{P_{2}}{P_{1}}=\left(\frac{T_{2}}{T_{1}}\right)^{4}=\left(\frac{2767+273}{487+273}\right)^{4}=4^{4}$

$\frac{P_{2}}{P_{1}}=\frac{P_{2}}{2P_{0}}=4^{4}$

$\Rightarrow \frac{P_{2}}{P_{0}}=2\times 4^{4}$

$\log_{2}\frac{P_{2}}{P_{0}}=\log_{2}[2\times 4^{4}]$

$=\log_{2}2+\log_{2}4^{4}$

$1+\log_{2}2^{8}=1+8=9$

• Advanced 2016 - Physics 2016
• Advanced 2016 - Chemistry 2016
• Advanced 2016 - Mathematics 2016